Rational Expressions and Equations 

Overview for Rational Expressions and Equations

 

Rational Expressions 

A look at rational expressions and solving equations from Paul's online notes

 

what's a rational equation? 

Learn about rational equations with examples and video

 

Intro to Rational Expressions 

Lessons on Rational expressions

 

Rational equations intro 

Lessons on rational equations from Khan Academy

 

Section 1-6 : Rational Expressions 

Lesson on Rational expressions and solving equations

Adding rational expression: unlike denominators 

Lessons on adding Rational Expressions

 

Adding & subtracting rational expressions: like denominators 

lessons from Khan Academy on adding and subtracting rational expressions with like denominators

 

Dividing Rational Expressions 

Dividing rational expressions from purple math

 

Multiplying & dividing rational expressions: monomials 

Lessons on Dividing and multiplying rational expressions

 

Multiplying Rational Expressions 

Learn how to multiply rational expressions

 

Multiplying Rational Expressions 

Overview on Multiplying Rational Expressions by cliffnotes

 

Multiplying Rational Expressions 

Pdf copy of Multiplying rational Numbers

 

Beginning Algebra 

book

Rational Expressions & Equations

 

A rational expression is nothing more than a fraction in which the numerator and/or the denominator are polynomials. 

 

A rational equation is an equation containing at least one rational expression.

 

Examples: 

Solve: 

Solution:

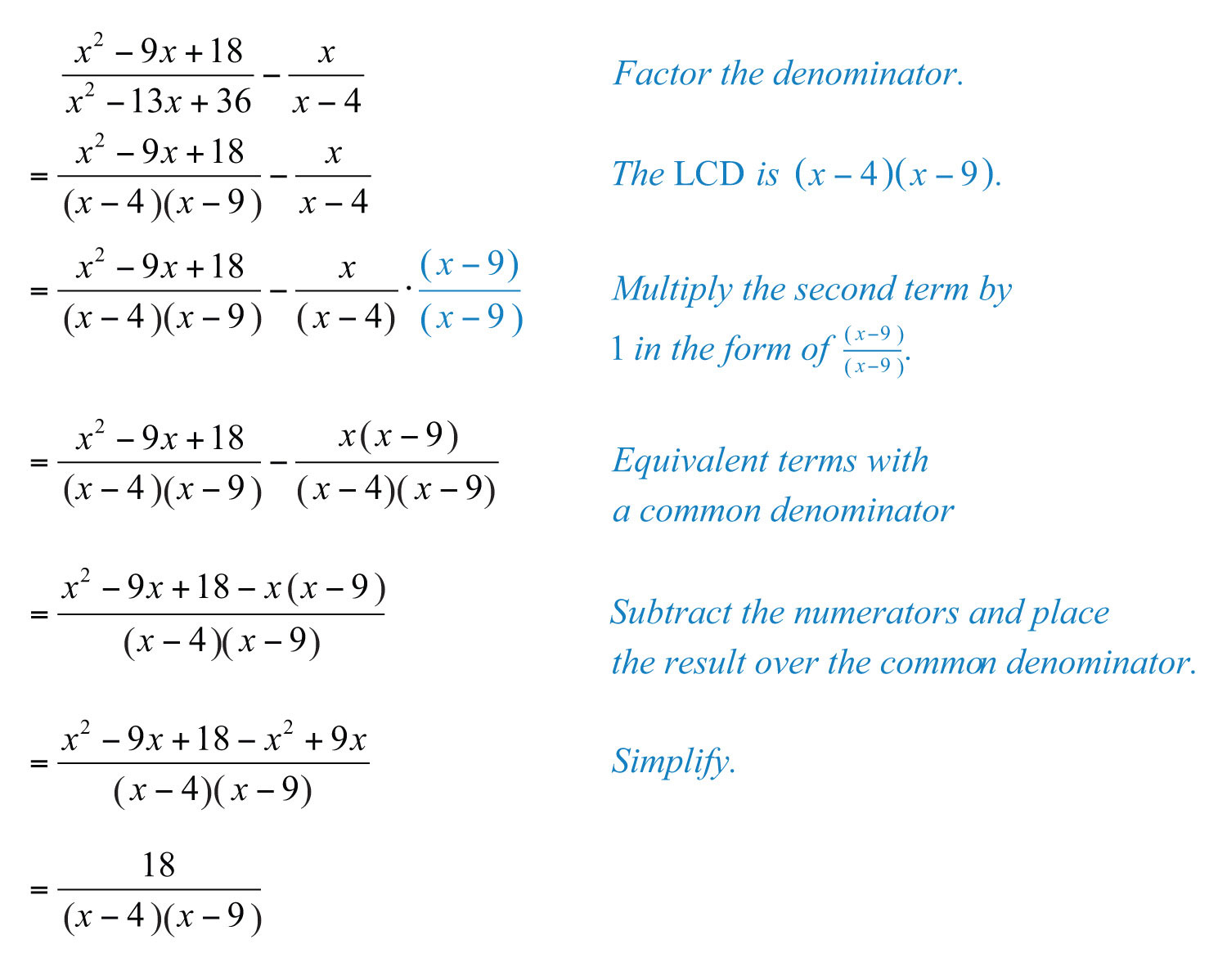
Step 1: Factor all denominators and determine the LCD.

The LCD is (x+2)(x+3).

Step 2: Identify the restrictions. In this case, they are x≠−2 and x≠−3.

Step 3: Multiply both sides of the equation by the LCD. Distribute carefully and then simplify.

Step 4: Solve the resulting equation. Here the result is a quadratic equation. Rewrite it in standard form, factor, and then set each factor equal to 0.

Step 5: Check for extraneous solutions. Always substitute into the original equation, or the factored equivalent. In this case, choose the factored equivalent to check:

Here −2 is an extraneous solution and is not included in the solution set. It is important to note that −2 is a restriction.

Answer: The solution is 4.

 

Problem retrieved from 

Saylor Academy: 7.5 Solving Rational Equations 

Lesson on Solving equations with Rational Expressions

Adding and Subtracting Rational Expressions

If the two rational expressions that you want to add or subtract have the same denominator you just add/subtract the numerators which each other.

Example:   

 

 

 

1. To add or subtract rational expressions with unlike denominators, first find the LCM of the denominator also called least common denominator , or LCD.
2. Write each expression using the LCD. Make sure each term has the LCD as its denominator.
3. Add or subtract the numerators.
4. Simplify as needed.

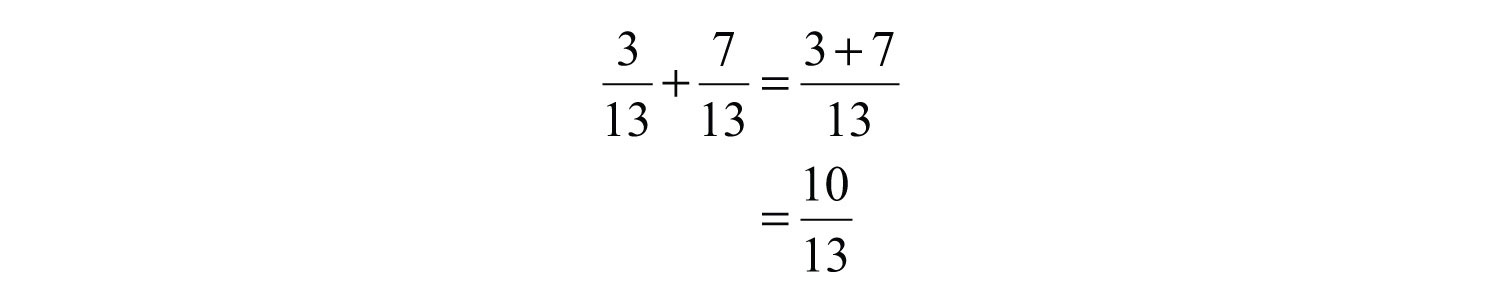
Example:

Multiplying & Dividing Rational Expressions   Multiplying:

 

Multiply the numerators together than multiply the denominators together.

Factor out the numerator and denominator 

make sure you check your constraints which means what numbers will make the denominator 0. 

Cancel or reduce the fractions. Remember that to reduce fractions; you can cancel something in the numerator with something in the denominator, but in order to cancel something in the numerator and denominator the two factors must be EXACTLY the same.

Example:

Simplify   .

This last answer could be either left in its factored form or multiplied out. If multiplied out, it becomes   

 

Dividing Rational Expressions:

When dividing a rational expression it is the same as multiplying by the reciprocal of the second set of numbers.

Change the division sign to a multiplication sign and flip (or reciprocate) the fraction after the division sign; essential you need to multiply by the reciprocal.

Cancel or reduce the fractions. Remember that to reduce fractions; you can cancel something in the numerator with something in the denominator, but in order to cancel something in the numerator and denominator the two factors must be EXACTLY the same.

Multiply across using the remaining factors to get final answer

Example: